How To Find The Height Of A Triangular Prism
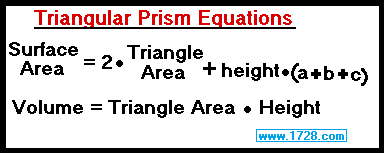
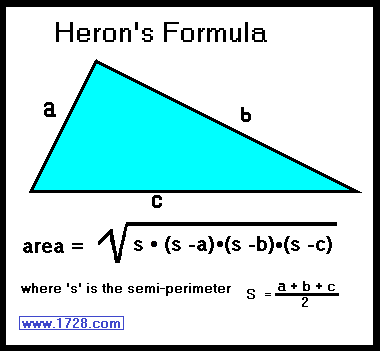
This computer computes a triangular prism'southward area and volume when the triangle sides and prism height have been input. The first matter to practise is to calculate the area of the triangular base of operations. Since nosotros know all three triangle sides, nosotros volition us Heron's Formula. Triangle Area = square root [9 * (9-v) * (9-6) * (9-7)] Since a triangular prism has ii triangles, the area of both triangles equals For the total prism expanse nosotros add up both areas:

A triangular prism is a geometric solid having 2 identical, parallel, triangular bases which are perpindicular to the iii rectangular faces.
In the in a higher place graphic, the light-green triangle is i of the bases and the ruby and blueish planes are two of the 3 faces.
The triangles can be whatever type of triangle (acute, correct or birdbrained).
It's great to have a estimator for computing triangular prisms, but let's see the mathematics of this.
A triangular prism has sides of v, half-dozen and 7 with a height of nine.
What is the surface area and volume of this prism?
Semi-Perimeter = 18 ÷ 2
Semi-Perimeter = 9
Triangle Area = square root [9 * 4 * iii * ii]
Triangle Area = square root (216)
Triangle Area = xiv.6969384567
2 * xiv.6969384567 = 29.3938769134
At present to summate the area of the iii rectangular faces:
Rectangular face area = 162
Total Area = 29.3938769134 + 162
Total Area = 191.3938769134
Volume = xiv.6969384567 * nine
Volume = 132.2724461103
Source: https://www.1728.org/triprism.htm
Posted by: paulinoalonese.blogspot.com


0 Response to "How To Find The Height Of A Triangular Prism"
Post a Comment